U.S. Department of Transportation
Federal Highway Administration
1200 New Jersey Avenue, SE
Washington, DC 20590
202-366-4000
Additional background information about curve advisory speed is provided in Bonneson et al. (5). The information in that report examines the objectives of curve signing and the challenges associated with establishing advisory speeds that are uniform among curves and consistent with driver expectation. That report also reviews the various criteria that have been used to set advisory speeds.
This part of the chapter examines the factors that influence the safety and operation of horizontal curves. The focus is on factors related to the curve's geometric design. The relationship between curve design and driver speed choice is described in Section 2.2.1, and the relationship between curve design and crash rate is explored in Section 2.2.2..
A review of the literature indicates that several variables can have an influence on curve speed. These variables include:
Of these variables, research indicates that the first five have the most significant effect on curve speed. Using data collected on rural highways in Texas, Bonneson et al. (5) developed a curve speed prediction model that includes sensitivity to these variables. The speeds predicted by this model are shown in Figure 1. The trends shown indicate that the average truck speed equals about 97 percent of the average passenger car speed.
Figure 1 - Effect of Radius, Tangent Speed, and Vehicle Type on Curve Speed
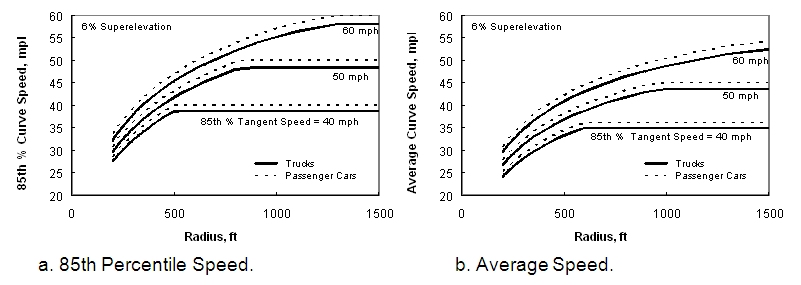
The trend lines in Figure 1 indicate that drivers on sharper curves slow from the tangent speed to an acceptable curve speed. The amount of speed reduction increases with decreasing radius. For curves with a 500 ft radius and a 60 mph tangent speed, the reduction is about 10 mph. In contrast, for a 1000 ft radius and 60 mph tangent speed, the reduction is only about 5 mph.
The effect of superelevation rate is not shown in Figure 1; however, the model indicates that curve speed increases about 1.0 mph for every 2.0 percent increase in superelevation.
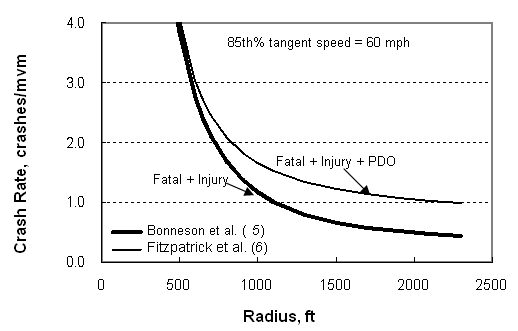
Bonneson et al. (5) examined the relationship between curve radius and crash rate using safety relationships documented in the literature (6, 7). These relationships are shown in Figure 2. In this figure crash rate is defined in terms of crashes per million vehicle miles (crashes/mvm). One trend line represents the combination of fatal and injury crashes. The other trend line represents the combination of fatal, injury, and property-damage-only (PDO) crashes.
The two trend lines in Figure 2 are in fairly good agreement. They indicate that the crash rate increases sharply for curves with a radius of less than 1000 ft. They also indicate that more crashes on sharper curves result in injury or fatality.
Based on the discussion in this and the previous sections, it is likely that the trends in Figure 2 are reflecting driver error while entering or traversing a curve. It is possible that some drivers are distracted or impaired and do not track the curve. It is also possible that some drivers detect the curve but do not correctly judge its sharpness. In both instances, traffic control devices have the potential to improve safety by making it easier for drivers to detect the curve and judge its sharpness.
Figure 2 – Curve Crash Rate as a Function of Radius
Most transportation agencies use a variety of traffic control devices to inform road users of a change in horizontal alignment. These devices include curve warning signs, delineation devices, and pavement markings. The focus of this part of the chapter is on curve warning signs; however, conditions where other traffic control devices may be helpful are also identified.
The MUTCD 2009 edition (3) identifies a variety of warning signs that can be used where the horizontal alignment changes in an unexpected or restrictive manner. These signs are shown in Figure 3. The recommended signs include:
Figure 3 - Horizontal Alignment Signs and Plaques (MUTCD 2009 Edition)

Compared to the MUTCD 2003 edition (8), the MUTCD 2009 edition (3) has three changes of the sign designation: 1) Combination Horizontal Alignment/Intersection Sign (W1-10) is expanded to include an additional series (W1-10a through W1-10e). This change offers more symbol designs to approximate the configuration of the intersecting roadway. 2) The Advisory Curve Speed Sign (W13-5 in MUTCD 2003 edition) is no longer used as a standard sign. 3) Combination Horizontal Alignment/Advisory Exit and Ramp Speed Signs (W13-6 and W13-7) are added into the horizontal alignment sign category.
The previous versions of the MUTCD criterion regarding the use of horizontal alignment warning signs can best be described as flexible. It encouraged engineers to base their signing decisions on engineering studies and judgment. However, this flexibility had the disadvantage of occasionally promoting inconsistencies in the application of traffic control devices. Inconsistent device application makes it difficult for drivers to develop reasonable expectancies and, consequently, promotes a disrespect of the device and mistrust of its message. The Advisory Speed plaque is perhaps one of best examples of the consequences of inconsistent sign usage. Research has found it to be among the more disrespected traffic control devices (9).
Research indicates that the inconsistent use of curve warning signs, especially those with an Advisory Speed plaque, may have lessened the average motorists' respect for the message the signs convey. On familiar highways, drivers come to learn that they can comfortably exceed the advisory speed for most curves. The concern is that these drivers may occasionally travel on roadways that are less familiar to them and where the advisory speed is posted at the maximum safe speed. These unfamiliar drivers may find themselves traveling too fast for conditions and experience a crash.
Only one report was found in the literature that documented the effect of horizontal curve signing with advisory speeds on safety. This 1968 report was a before-after study by Hammer (10) of the installation of warning signs in advance of several curves. He found that the implementation of advance horizontal alignment signs reduced crashes by 18 percent. He also found that the combined use of advance signing with an Advisory Speed plaque reduced crashes by a total of 22 percent.
Research by Ritchie (11) examined driver response to the Curve sign and the Advisory Speed plaque. He found that average curve speeds exceeded the advisory speed when the advisory speed was less than 45 mph.The amount by which the average speed exceeded the advisory speed increased with reduced advisory speeds. Thus, for an advisory speed of 40 mph, the average speed exceeded the advisory speed by only 2 mph (i.e., the average speed was 42 mph); however, for an advisory speed of 20 mph, the average speed exceeded the advisory speed by 10 mph.
The findings of this review are consistent with those noted in Section 2.2.2. Specifically, drivers do not appear to be responding to the Advisory Speed plaque by reducing their speed to the advisory speed. Hence, measuring the amount of speed reduction may be of limited value in assessing the effect this sign has on safety. However, these findings suggest that advance information about an upcoming curve, as provided by a curve warning sign, may heighten driver awareness of the curve, but it does not cause them to slow considerably. Perhaps this heightened awareness produced the safety benefit found by Hammer (10).
This part of the chapter provides an overview of the Curve Advisory Speed (CAS) software. This spreadsheet was developed to automate the procedures and guidelines described in this handbook. The background for the development of the equations in this spreadsheet is documented in an earlier research report by Bonneson et al. (5). The current Curve Advisory Speed (CAS) software accommodates several methods for establishing advisory speeds and follows the curve signing criterion according to the MUTCD 2009 edition (3).
The "Analysis" tab worksheet contains the curve advisory speed calculations. This worksheet is shown in Figure 4. Six (6) columns are provided in the worksheet. One column is used for each curve being evaluated.
Figure 4 – Curve Advisory Speed (CAS) Software Analysis Worksheet
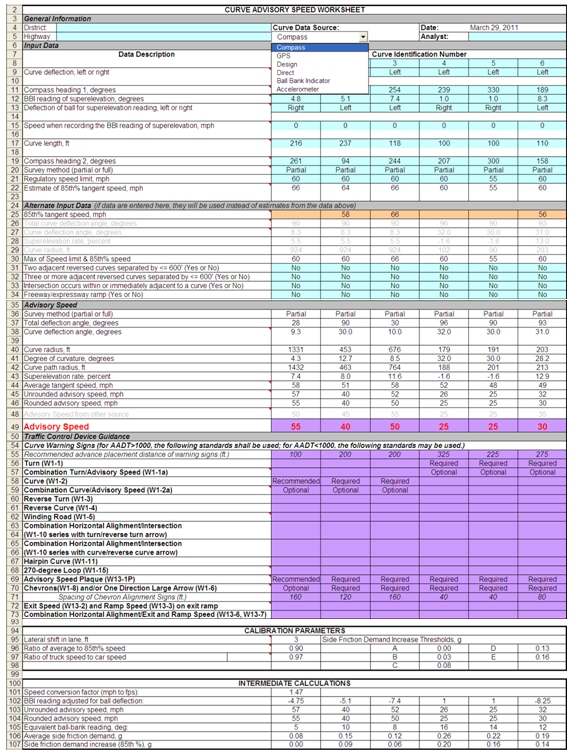
The spreadsheet can be used with six types of input data. One method is based on data obtained from a survey of the curve using the Compass Method. This method is described in Chapter 3. The drop-down list located in cell F5 is used to specify this method by selecting "Compass," as shown in Figure 4. The data from the Compass Method are entered in the cells that have a light blue shaded background in the rows 9 through 21 and are designated "input data" cells. If the 85th percentile tangent speed is not known, then this cell should be left blank, and row 25 and the estimate in the row 22 (or the speed limit in the row 21, whichever is larger) will be used as the 85th percentile speed. The cell in the row 25 (orange shaded) is optional to input the 85th percentile speed of free-flowing passenger cars on the tangent prior to the curve. The information in the rows 31 to 34 (light blue shaded) are required to account for special roadway configuration.
The second and third methods of data input are based on describing the curve deflection angle, superelevation rate, and radius. These data can be obtained from the GPS Method or the Design Method, as described in Chapter 3. The use of either of these methods is specified with the drop-down list located in cell F5 by selecting "GPS" or "Design." The data from the GPS Method or the Design Method are entered in the light blue shaded cells in the row 21 and 26 through 29. If the 85th percentile tangent speed is not known, then this cell should be left blank, and the estimate in the row 22 (or the speed limit in the row 21, whichever is larger) will be used as the 85th percentile speed. The cell in the row 25 (orange shaded) is optional to input the 85th percentile speed of free-flowing passenger cars on the tangent prior to the curve. The information in the rows 31 to 34 (light blue shaded) are required to account for special roadway configuration.
The fourth, fifth and sixth methods of data input are based on other methods, such as the Direct Method, the Ball-Bank Indicator Method, and the Accelerometer Method, as described in Chapter 3. The use of any of these methods is specified with the drop-down list located in cell F5 by selecting "Direct," "Ball-Bank Indicator," or "Accelerometer." The advisory speed established by the other method is entered directly in the light blue shaded cells in the row 48. The cell in the row 21 (regulatory speed limit) is required to apply MUTCD 2009 edition (3) signing criteria. The information in the rows 31 to 34 (light blue shaded) are required to account for special roadway configuration. It is optional to input the 85th percentile speed of free-flowing passenger cars on the tangent prior to the curve in the cell in row the 25 (orange shaded). If the 85th percentile speed is not known, then this cell should be left blank, and the estimate in the row 22 (or the speed limit in the row 21, whichever is larger) will be used as the 85th percentile speed. The information, total curve deflection angle, in the cell in the row 26 (light blue shaded) is used for curve warning sign determination process.
The cells in the rows 36 to 46, which do not have background shading, contain equations for the first three input methods. The basis for each equation is documented in Bonneson et al. (5). These equations document the analysis of advisory speed for each of the six curves. The purple shaded cell in the row 49 is the advisory speed established by the applied method.
The purple shaded cells in the rows 54 to 73 of the spreadsheet document the traffic control device guidance. The criterion described in Chapter 4 of this manual and the MUTCD 2009 edition (3) are used to calculate the information that is summarized in this section of the spreadsheet. If cells are blank in this section of the spreadsheet then the traffic control device for that column is not required for that curve.
The cells in the rows 94 to 107 of the spreadsheet contain many parameters that control the computations. The default model used for calculating the advisory speed is based on the average truck speed (5). The corresponding formulation is as follows:
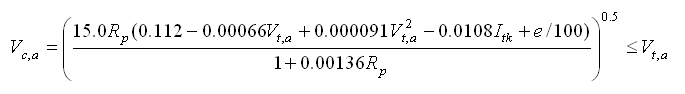
where,
Rp = travel path radius, ft;
Vc,85 = 85th percentile curve speed, mph;
Vt,85 = 85th percentile tangent speed, mph;
Vc,a = average curve speed, mph;
Vt,a = average tangent speed, mph;
e = superelevation rate, percent; and
Itk = indicator variable for trucks (= 1.0 if model is used to predict truck speed; 0.0 otherwise)
Note that the default model used in the Curve Advisory Speed (CAS) software assumes to use the estimated average truck speed to establish the advisory speed for a particular curve. This is a conservative approach proposed by TTI to ensure safety. However, there is no consensus or agreement among various transportation practitioners on whether to use passenger car vs. truck and average speeds vs. 85th percentile speeds as the criteria to establish advisory speeds. Therefore, it is suggested, before modifying these parameters in the spreadsheet, to carefully read through the report by Bonneson et al. (5) and think about if the default assumption matches your engineering experience and judgment.
If other criteria are chosen to establish the advisory speed, the parameters can be modified. For example, if the 85th percentile speed is preferred to be used as the advisory speed, the formulation shall be changed to the following equation.
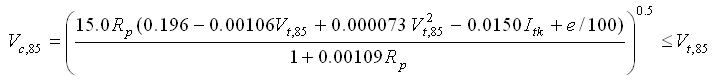
The formulation is changed through the modification in the cell in the row 103.
If the passenger car speed is preferred to be used to establish the advisory speed, due to low volume of trucks or low truck crash rate, the value in the cell in the row 97 shall be changed to 1.0 and Itk in the formulation shall be set to 0.0.
